News
Article
Pharmaceutical Technology
Small-Angle X-ray Scattering for Pharmaceutical Applications
The author describes the development of small-angle X-ray scattering and analyzes its advantages in the characterization of drug-delivery systems and large molecules. This article is part of a special Analytical Technology issue.
Small-angle X-ray scattering (SAXS) offers various ways to characterize drug-delivery systems and large molecules. Understanding the structure of drug-delivery systems and large molecules at a molecular level is a crucial step in designing drugs and drug-delivery systems alike.
The SAXS technique can provide insights into structures in the 1–100 nm range. SAXS requires little or no sample preparation and enables scientists to run experiments at or close to in vivo conditions.
Historical perspective
Röntgen discovered X-rays in 1895. In 1912, Laue discovered the diffraction of X-rays by crystals (1). Guinier's work in late 1939 led to the main principles of SAXS (2). In the 1940s and 1950s, Otto Kratky investigated X-ray diffraction at small angles as a technique for the structural analysis of macromolecules. He developed the SAXS method into a powerful tool for structural research, particularly in the field of polymers and molecular biology (3). Considered one of the fathers of SAXS, Kratky founded the Institute for Physical Chemistry in Graz, Austria, which became an early center for this technique. The institute led to many advances in SAXS such as the first commercial instrument for SAXS.
Early SAXS experiments took place in laboratories. In the 1970s, the availability of synchrotrons and high-intensity synchrotron radiation helped bring the technique to prominence. In recent years, technical advances have made laboratory-based SAXS instruments attractive again.
SAXS basics
SAXS is a form of X-ray diffraction that focuses on small scattering angles. Scattering intensities at large angles (i.e., wide-angle X-ray scattering) contains information about small objects such as crystalline structures. Small angles contain information about large objects such as particles, macromolecules, and micelles. Figure 1 shows this inverse relation. This article will use the terms "particles," "macromolecules," and "micelles" interchangeably.
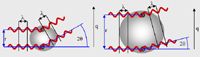
Figure 1: Positive interference of two spherical waves from an electron pair can be seen (left) at larger angles if the electrons are close to each other (e.g., in a crystalline structure) and (right) at smaller angles if the distance is greater (e.g., in a macromolecule). (ALL IMAGES ARE COURTESY OF THE AUTHOR)
Although its name contains the word "angle," the scattering vector q is common for SAXS. Also known as momentum transfer, q reflects the process in which the X-ray photons transfer energy to the electrons with which they interact. The following equation describes the relation of the length of the scattering vector q and the scattering angle 2θ commonly used in other X-ray diffraction methods (4):
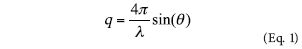
The measured intensity I as a function of the scattering vector q is given in the following equation:
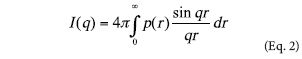
The pair-distance distribution function p(r) in Equation 2 is the geometrical representation of the object in the beam. p(r) maps the distances of all electron pairs inside the particle. The scattering intensity I and the geometrical representation p(r) are related by Fourier transform.
However, representing a three-dimensional (3D) object with a one-dimensional distribution function necessarily omits some information. Converting p(r) into a three-dimensional object becomes difficult and requires additional constraints by the scientist such as connectedness or compactness.
Technical concepts
The main technical challenges of SAXS are to separate the strong primary X-ray beam from the weak scattering information close to the primary X-ray beam and to increase the intensity of the scattered X-rays.
Scientists have used different concepts to achieve these goals. However, all SAXS instruments feature an X-ray source, a collimation system focusing optics, a sample holder, a beam stop, and a detector (see Figure 2).
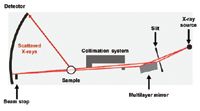
Figure 2: A typical setup for a small-angle X-ray scattering instrument includes a source, collimation system with optics (i.e., mirror), sample holder, and detector. The area that the beam enters after passing the mirror is typically evacuated to reduce unwanted scattering from gas molecules.
X-ray sources
To date, copper anodes, producing Cu–Kα radiation, have been the best compromise between sensitivity and absorption. The following three technologies are currently in use:
- Sealed-tube sources
- Rotating-anode sources
- Microfocus sources.
In sealed-tube sources, the electrons are accelerated toward a fixed anode. Part of the energy is emitted as X-rays, but the majority is converted to heat. In sealed-tube sources, the anode is fixed, so a limited amount of energy can be dissipated before the copper melts. Rotating-anode sources exceed this limit and avoid local overheating (5).
Microfocus sources use electromagnetic lenses to focus the electron beam to a fine point, thus producing a high-brilliance X-ray beam.
Scientists typically consider operating costs, energy consumption, and flexibility when choosing an X-ray source. They also must determine whether the source will match with other components of the SAXS system.
X-ray optics and collimation systems
Focusing optics increase the photon flux through the sample. Visible-light lenses and electromagnetic fields such as electron beams cannot focus X-rays. Multilayer mirrors reflect X-rays on a layered surface under the Bragg condition (see Equation 3). Depending on the layers' thickness, a certain wavelength positively interferes (i.e., reflects) at a specific angle (5).
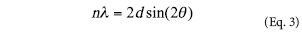
By reflecting certain wavelengths at certain angles, multilayor mirrors can produce monochromatic X-rays. The mirror's curvature produces the desired beam focus, typically parallel or converging.
The monochrome and focused beam then travel through the collimation system to produce a precise beam profile. The following two collimation methods are common:
- Point collimation (i.e., a pinhole system)
- Line collimation (i.e., a slit system).
Point-collimation systems send a point-shaped beam through a series of three pinholes. The first two pinholes produce a parallel beam, and the third pinhole blocks unwanted scattering produced by previous pinholes.
Line-collimation systems use a line-shaped beam and employ slits instead of pinholes. The block collimator, a compact and effective design to eliminate parasitic scattering by the collimation system itself, was suggested by Kratky (4).
Line collimation increases the photon flux through the sample by replacing a single point-shaped beam with the equivalent of many such points arranged in a line. The increase in intensity comes at the cost of a smearing effect on the scattering image (4). Figure 3 shows the effects of line collimation.
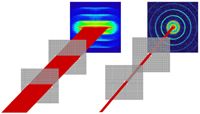
Figure 3: Scattering patterns of silver behenate. The liquid crystalline structure with clear concentric rings in the case of a point-shaped beam profile (i.e., point collimation) (right) becomes smeared when using a line-shaped beam (i.e., line collimation) (left). The line-collimated experiment yields higher intensity. Reversing the smearing effect requires knowledge of the beam profile and position.
Desmearing data is a mathematical operation that requires knowledge of the beam profile that causes the smearing effect (6–8). New technologies report the beam profile and position so that desmearing becomes a simple and precise routine, thus alleviating earlier concerns (9, 10).
Detection systems
X-ray detectors must be able to capture faint scattering signals. Scientists currently use the following technologies (5):
- Imaging-plate detectors
- Charged-coupled device detectors
- Wire detectors
- Diode-array detectors.
The pixel resolution determines the required distance between the detector and the sample. High-resolution detectors can be brought closer to the sample, but low-resolution detectors should be positioned further away from the sample.
The intensity of the scattered X-rays decreases with as distance increases, according to the following quadratic law:
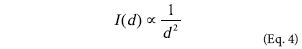
A detector with a distance 2d to the sample receives only 25% of the intensity of a detector with a distance d to the sample at the same photon flux.
Typically, a beamstop protects the detector from exposure to the direct beam, which is several magnitudes more intense than the scattered X-rays.
Experimental setup
Dilute systems allow the analysis of particlate and intraparticlate structure. Interaction between particles can be observed with highly concentrated samples.
Scientists place the prepared measuring sample into a quartz capillary or a cell with Kapton (DuPont, Wilmington, DE) or polycarbonate windows.
All electrons in the path of the beam interact with the X-ray photons. Application of a vacuum prevents the unwanted scattering by gas molecules. The contributions of capillary material, windows, and the solvent are significant. These factors need to be considered separately.
The majority of the scattered intensity comes from electrons that are not part of the molecule or particle of interest. To remove this unwanted scattering, scientists conduct a second measurement with identical setup, but without the protein or particle in solution. The difference between the two measurements is the contribution of the molecule or sample under investigation. This process is called background subtraction.
Exposure times. For the SAXS experiment, the sample holder, containing the sample to be measured is inserted into the camera system, between the collimation system and detector. The shutter of the X-ray source is then opened for a certain amount of time, thus exposing the sample to X-rays.
The exposure time depends on the X-ray photon flux through the sample, the sample-detector distance, and the efficiency of the detector at converting the scattered X-ray photons into measurable electrical signals. The exposure time typically ranges from fractions of a second to seconds on a synchrotron beam line and from minutes to hours on laboratory-based SAXS systems.
Primary data handling. Upon completion of the experiment, the resulting intensity map is analyzed. In the first step, the scattering image is reduced to a scattering curve as a function of the scattering vector q by integrating the image data over a pie slice area (i.e., in point collimation) or box area (i.e., in line collimation). The same is done for the background experiment.
Next, scientists subtract the background curve from the sample measurement. The remaining curve is then ready for further evaluation (see Figure 4).
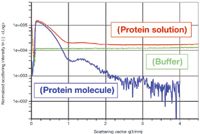
Figure 4: Scattering image of a protein solution (red) and buffer (green). Subtracting the buffer from the solution yields the information of the actual protein molecule (blue).
Analysis and evaluation
After background subtraction and desmearing (if applicable) the data are ready for the following analytical steps:
- Determining the radius of dyration
- Calculating the surface–volume ratio
- Finding the peak spacing for determining liquid crystalline structure
- Indirect Fourier transform
- Fitting to theoretical models
- Deconvolution of the radial electron-density profile
- Ab initio 3D shape and domain modeling.
Applications
SAXS has proven particularly useful in several pharmaceutical applications. This section will describe the most important of them.
Functionalization of self-assembled structures. Self-assembled structures have provoked considerable interest because they can lead to functional materials with nanoscale structures (11–13). SAXS can determine key relationships between nanostructures and their functions.
Scientists often use micelles, self-assemblies of amphiphilic molecules, to solubilize water-insoluble functional substances. Lyotropic liquid crystals in lamellar and reversed-hexagonal phases are used for emulsion-type products. Liposomes or vesicles consisting of phospholipids or synthetic surfactant bilayers play an essential role and act as nanocapsules. Polymer gels are used in various products, including drug carriers.
Pharmaceutical materials. Drug-delivery systems transport drugs to affected parts of the body. Nanocarriers, typically in the size range of 20 to 100 nm, provide the required amount of drugs promptly to a specifically affected part with pinpoint accuracy. Various self-assembly systems (e.g., micelles, microemulsions, liposomes, cubosomes, and polymer-gel nanoparticles) have been tested as drug carriers.
Liquid crystals. Lytropics are important for biological systems. The SAXS method provides structural information about heterogeneities, aggregate ordering, size, shape, separation, and intermolecular spacing within the aggregate stack and helps scientists study the interdependence of morphology and phase behavior. The applications of liquid crystals include biological membranes (e.g., drug-delivery carriers).
Mesoporous materials. Mesoporous materials have pore apertures similar in size to small biological molecules. Mesoporous materials with a narrow pore-size distribution may thus be useful as hosts, supports, catalysts, and separation media for these molecules. The pore-size distribution can be determined with SAXS.
Membranes. The functionality of biological membranes depends on the geometrical and chemical properties of the amphiphilic molecules of the membrane walls. Membrane parameters such as the electron-density profile or flexibility affect the membrane's functionality. Permeability and the tendency to reorganize into micelles, lamellar stacks, or vesicles strongly depend on the internal arrangement of the molecules in the bilayers.
The phospholipid-bilayer membranes can be studied with the SAXS for electron density, thickness, repeat distance of lamellae and stacks, number of layers, packing, and flexibility parameters.
Proteins in solution. Proteins are complex macromolecules. Many diseases are linked to protein misfolding. Structure changes may happen as a function of time, pH, ionic strength, and changes in various solution conditions. SAXS helps identify structural states and changes of biological macromolecules and helps correlate these changes to their biological functions. Over the past decades, 3-D structures of a vast number of biological molecules have been determined using X-ray crystallography and nuclear-magnetic resonance (NMR) (14). However, these high-resolution methods have their own limitations.
Structure determination by X-ray crystallography requires high-quality protein crystals that are complex and costly to produce. NMR allows structures in solution to be studied, but the size of the protein typically accessible by NMR is still much smaller than that of X-ray crystallography.
Some SAXS instruments allow simultaneous measurements of small and wide-angle X-ray scattering from proteins in solution. SAXS successfully determines the ternary and quaternary structures by investigating the overall size and shape. The technique has achieved considerable success in restoring 3-D structures of proteins from the scattering patterns. Experimental setups with wide simultaneous and continuous q-ranges directly probe distance correlations on length scales that are small compared with the overall protein dimensions. The setups may contain rich information about fine-structure details of proteins in solution (15–18). Scattering data in the higher q region is sensitive to protein conformation states (i.e., secondary structures and their packing) and also enables scattering patterns to be compared quantitatively with calculated patterns from detailed structure models. It can be used as an additional information input for the evaluation of NMR data as well (19, 20).
Protein crystallization. To understand and predict protein crystallization from solutions have been paramount tasks for years. The SAXS method's sensitivity helps it detect aggregate structures at an early stage and therefore allows it to indicate the optimum conditions for the onset of protein crystallization.
Lipoproteins. Several diseases are associated with changes in the concentration of blood lipoproteins. SAXS performs fast and precise measurements of lipoprotein particles using small amounts of sample. Its ability to measure all fractions of lipoproteins simultaneously makes SAXS a cost-effective and convenient method for lipoprotein analysis in scientific studies and medical practices.
Carbohydrates. The SAXS method is useful in investigating native starch, its structure, and changes in the structure. The technique also helps measure lamellar repeat distance, fractional lamellar crystallinity, width of the distribution of lamellar sizes, and the number of semicrystalline repeats within each growth ring.
Conclusion
Small-angle X-ray scattering (SAXS) has emerged as an essential tool for structural analysis at length scales as large as 100 nm. The SAXS method yields information not only on particle size and shape, but also on the internal structure and radial electron density profiles of disordered and partially ordered systems.
In the past, the experimental data only enabled the direct determination of overall particle parameters (e.g., mean radius of gyration, particle symmetry, surface per volume) of the macromolecules. In terms of 3D models, the analysis was limited to simple geometrical bodies (e.g., spherical, cylindrical, lamellar) or combinations of them.
The 1990s brought a breakthrough in SAXS data analysis methods that allowed ab initio shape and domain-structure determination and detailed modeling of macromolecular complexes using rigid body refinement (21–23). In dense systems, the scattered intensity is a combination of single-particle scattering (i.e., form factor) and interparticle scattering (i.e., structure factor). The generalized indirect Fourier transform method, one of the most recent developments in data-evaluation programs, allows the interpretation of such scattering data (24). Information about interparticle interactions in dense systems can be deduced by analyzing the structure factor with various potential models assuming repulsive or attractive interactions. SAXS has become a powerful tool for pharmaceutical and biotechnology applications.
Acknowledgements
The author would like to thank Heimo Schnablegger, product manager of X-ray structure analysis at Anton Paar, for his comments and suggestions.
Gerd Langenbucher is a product manager for Physica rheometers and Anton Paar SAXSess X-ray structure analysis at Anton Paar USA, 10215 Timber Ridge Dr., Ashland, VA 23005, tel. 804.550.1051, ext. 126, fax 804.550.1057, gerd.langenbucher@anton-paar.com
References
1. Fifty Years of X-ray Diffraction, P.P. Ewald, Ed. (A. Oosthoek's Uitgeversmaatschappij, Utrecht, 1962).
2. M. Lambert, Acta Crystallogr. 57 (1), 1–3 (2001).
3. J. Schurz, Colloid Polym. Sci. 273 (10), 994–995 (1995).
4. Small Angle X-ray Scattering, O. Glatter and O. Kratky, Eds. (Academic Press, London, 1982) 4f.
5. H. Schnablegger and Y. Singh, "A Practical Guide to SAXS," (Anton Paar, Graz, Austria, 2006).
6. J. Lake, Acta Crystallogr. 23 (2), 191 (1967).
7. G.R. Strobl, Acta Crystallogr. A 26 (3), 367–375 (1970).
8. M. Soliman, B.J. Jungnickel, and E. Meister, Acta Crystallogr., A, Found. 54 (5), 675–681 (1998).
9. C. Jeffries et al., J. Mol. Biol. 377 (4), 1186–1199 (2008).
10. H. Schnablegger, "Is Smearing An Issue?" (Anton Paar Graz, Austria, 2007).
11. M. Muthukumar, C.K. Ober, and E.L. Thomas, Science 277 (5330), 1225–1232 (1997).
12. G.M. Whitesides, J.P. Mathias, and C.T. Seto, Science 254 (5036), 1312–1319 (1991).
13. G.M. Whitesides and B. Crzybowski, Science 295 (5552), 2418–2421 (2002).
14. A.M. Edwards et al., Nat. Struct. Biol. 7 (11), 970–972 (2000).
15. R.F. Fischetti et al., J. Synchrotron Radiat. 10 (5), 398–404 (2003).
16. M. Hirai et al., J. Synchrotron Radiat. 9 (4), 202–205 (2002).
17. D.M. Tiede, R. Zhang, and S. Seifert, Biochemistry 41, 6605–6614 (2002).
18. R. Zhang, P. Thiyagarajan, and D.M. Tiede, J. Appl. Crystallogr. 33 (3), 565–568 (2000).
19. D.I. Svergun and M.H.J. Koch, Rep. Prog. Phys. 66 (10), 1735–1782 (2003).
20. J. Trewhella and J.K. Krueger, Methods Mol. Biol. 173, 137–159 (2002).
21. P. Chacon et al., Biophys. J. 74 (6), 2760–2775 (1998).
22. P. Chacon et al., J. Mol. Biol. 299 (5), 1289–1302 (2000).
23. D. Svergun, Biophys. J. 76 (6), 2879–2886 (1999), erratum in 77, (5), 2896–2896 (1999).
24. G. Fritz, A. Bergmann, and O. Glatter, J. Chem. Phys. 113 (21), 9733–9740 (2000).

Newsletter
Get the essential updates shaping the future of pharma manufacturing and compliance—subscribe today to Pharmaceutical Technology and never miss a breakthrough.