A Comparison of Three Extrusion Systems

Multiparticulate oral dosage forms have gained considerable popularity since their market introduction because of their numerous pharmaceutical and technological advantages and their suitability for pediatric use (1–3). From a pharmaceutical point of view, pellets can reduce the variations in gastric drug levels, reduce inter- and intraindividual variations, minimize side effects and high local concentrations, and allow modified-release kinetics. They also enable otherwise incompatible active ingredients to be combined in one dosage form. In pediatrics, pellets offer the advantages of administration with food and the possibility of adjusting doses according to the child's body mass. The major technological advantage of pellets is their capacity to be adapted to successful coating operations (e.g., for a sustainedrelease diltiazem formulation). Furthermore, pellets enhance flow properties during capsule filling, provide a narrow size distribution of particles, and offer low friability.

Among the different methods to produce pellets, the process of extrusion–spheronization is of particular interest (1, 3). Extrusion–spheronization is a semicontinuous process organized in five unit operations: blending, wet granulation, extrusion, spheronization, and drying (4). This process, fast and robust, limits the use of organic solvent and enables drug loading as high as 90%, depending on the active properties, in the mixture. When used to make finished products, extrusion–spheronization produces well-densified pellets, offers a narrow particle-size distribution, yields low friability, ensures regular sphericity, and maintains good flow properties.

The properties of the final product depend on the physicochemical properties of the raw materials and the amount of each component in the formulation (5). Various process variables also affect the quality of the pellets. These variables include the type and quantity of solvent added to the powder mixture; mixing time and speed; type of extruder, design of the screen, and rate of extrusion; spheronization speed, time, load, and plate design; and drying rate and time (2–4).

Because various extruder designs are available to prepare extrudates from the wet mass, numerous authors have studied the effect of different extruders on process characteristics and pellet properties. Extruders can be divided into three main categories, according to their feed mechanism: screwfeed (i.e., single- or twin-screw), gravity-feed (i.e., sieve, gear, cylinder, and basket), and ram extruders (3, 4).

Few studies compared any extruders with the ram extruder to provide rheological information and to validate the latter extruder's prediction power. Some authors drew parallels between a ram extruder and a gear extruder or a cylinder extruder, in terms of extrusion characteristics and pellet properties (6–8). Others compared a twin-screw extruder with a gear extruder or with a rotaring-die press by examining the extrusion process and pellet quality (9, 10). A roll-press cylinder also was compared with a basket and a single-screw extruder in terms of pellet characteristics (11). Differences in process and pellet properties between a cylinder, an axial single-screw, a radial basket screen, and a ram extruder were studied (12, 13). The authors underlined great differences between the feeding systems, thus demonstrating that it was not always possible to transfer a formulation directly from one type of extruder to another.

Few authors have compared various extrusion systems with the same extrusion-feed mechanism. This approach seems to be particularly attractive for screw-feed extruders, which can be classified in three categories according to the design of the screen (i.e., axial, dome, and radial) (3). The comparative influence of radial and axial single-screw extruders on the extrusion process characteristics and on the quality of final product was studied using various formulations (14–16). Other authors compared two twin-screw axial extruders for continuous granulation on pellet quality (17). Nevertheless, no author has compared dome technology to the two other screw-feeding technologies. Few authors have studied the dome extruder as a simple tool for extrusion (18–21).

Numerous authors showed the influence of water quantity on extrudate or pellet properties when using a ram extruder, a gravity-feed extruder, a single-screw extruder, or a twin-screw extruder (5, 9, 11, 22–33). Other authors showed that extrusion speed influenced extrudate or pellet quality in ram extruders, gravity-feed extruders, single-screw extruders, and twin-screw extruders (27, 31, 33–37). Several authors showed extrusion systems' different sensitivities to water content and to extrusion speed (10–14, 17).

In this context, studying the influence of water quantity and extrusion speed is an interesting way to highlight differences between extrusion systems. The authors aimed to compare the three systems of single-screw extrusion—radial, dome, and axial—in terms of productivity and the properties of pellets created by extrusion–spheronization. To highlight differences between the three extrusion systems, various levels of water content and extrusion speeds were tested. A majority of previous studies indicated that these two parameters have great influence. The authors set up a response surface design of experiments to reveal the variables' influence and to identify the type of extruder that yielded the best productivity and pellet quality.

Materials and methods

Raw materials. Pellets were prepared from a binary mixture of a drug substance (DS) and microcrystalline cellulose (MCC). The DS, an antidepressant supplied by Pierre Fabre Research Institute, is highly soluble in water (1250 g/L, median drug particle size: 14 μm, dispersion: 1.5). MCC (Avicel PH101, median drug particle size: 50 μm, FMC Biopolymer, Philadelphia) is insoluble in water. The ratio of DS to MCC was 36:64 (% w/w) in this study. Purified water was used at different concentrations as liquid binder.

Experimental design. A response surface design of experiments was built with Design Expert software version 7.0.0 (Stat-Ease, Minneapolis). The mathematical model targeted for each response studied was a quadratic model with firstorder interactions. The authors studied three factors: percent water quantity (A), extrusion speed (B), and extrusion system (C). To analyze the results, the extruder system was included as a qualitative factor, whereas water quantity and extrusion speed were continuous factors.

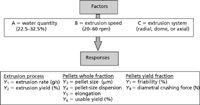
Figure 1: Factors and responses of the experimental design.

The levels of the continuous factors were determined by preliminary trials. Five levels of water quantity were tested in the range of 22.5–32.5%. According to preliminary tests, it was impossible to create pellets with water quantities beyond these limits. Three levels of extrusion speed were tested in the range of 20–60 rpm. Radial, dome, and axial extrusion systems were tested. All other experimental conditions were kept constant. Two replicates of the median conditions (i.e., 27.5% water and 40 rpm extrusion speed) were run for each extruder type. The experimental design comprised 27 experiments. All factor-level combinations were carried out in a randomized order. Figure 1 summarizes factors and responses selected for the design of experiments.

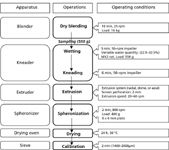
Figure 2: Pellet-manufacturing flow chart.

Pellet preparation. MCC pellets containing 36% DS were made by extrusion–spheronization. Dry blending of MCC and DS was a common step to all the batches. After blending, 550 g of dry mix were sampled for subsequent steps. Wetting and granulation were performed in an Aoustin kneader (RPA Process, Nanterre, France). The wet mass obtained was extruded in an MG-55 extruder (FujiPaudal, Osaka). The extrudates were then spheronized in a Multi Bowl Spheronizer 250 (Caleva, Sturminster Newton, UK) and the pellets obtained were dried in a UT6120 drying oven (Heraeus, Hanau, Germany). Process conditions were fixed according to the flow chart shown in Figure 2. The authors used the MG-55 single-screw extruder to test the three extrusion configurations (see Figure 3). The axial system incorporated a flat end-plate screen perpendicular to the end of the screw. The dome system had an arch-shaped endplate screen at the end of the screw. The extrudates were ejected out of the screens at the end of the axial and dome extruders. In the radial system, the screen was placed around the screw so that the extrudates were ejected perpendicular to the motion of the screw. A die of 2 mm in diameter was used in this study to allow comparisons. The extruders' total open areas also were different. The screen thickness of the radial, dome, and axial systems presented 52.4, 22.7, and 15.5% open area and a 1-, 1.2-, and 2-mm screen thickness, respectively.

Figure 3: Extrusion of the wet mass in radial, dome, and axial extrusion systems.

Characterization. Particle-size distribution of the pellets was determined with a laser diffractometer (Mastersizer 2000, Malvern Instruments, Malvern, UK) equipped with a dry-powder dispersing system (Scirocco 2000, Malvern Instruments, Malvern, UK) using a dispersion pressure of 1 bar. Particle-size distributions were characterized by their volume median diameter d_0.5 (μm) and their pellet-size dispersion d_g. The pellet-size dispersion was calculated from d_0.5, d_0.1, and d_0.9 according to the following equation:

where d_0.1 and d_0.9 values are the particle diameters corresponding to 10% and 90% of the cumulative distribution, respectively. The materials were passed through a 2000-μm sieve before the measurement, and the pellet fraction over this size was re-entered in the results. The pellets' mean size should be smaller than the diameter of the extruder die (i.e., 2 mm) because of the densification and water evaporation that occurred during the spheronization and drying stages. The pellets' size dispersion was required to be narrow as possible, expressed by a low (i.e., < 3) d_g value, to facilitate coating or capsule-filling operations.

Pellet morphology was evaluated by microscopic observations (Stereo Microscope SMZ-168-TL and Moticam 2300 microscopes, Motic Microscopes, Xiamen, China). The morphological analysis of pellets was performed by means of a particle-image analyzer (Morphologi G2, Malvern Instruments). Analysis was carried out on roughly 300 pellets. Numerous parameters can be used to describe pellets' shape. For the current study, pellet elongation (E) was calculated according to the following formula:

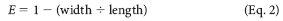
Because the pellets eventually are filled into capsules, they should present good flow characteristics and be as spherical as possible. For a perfect disk, the value of the elongation factor equals 0. It is desirable to obtain pellets with the least elongation possible.

The pellets' whole fraction was sieved on 1400–2000-μm sieves (Retsch, Haan, Germany) for 2 min, at a frequency of 60 Hz with an amplitude of 1 mm. The 1400–2000-μm fraction of pellets was considered the usable fraction. The authors used this fraction for pellets characterizations to eliminate the effect of size on the pellets' mechanical properties. The usable yield had to be as high as possible.

Friability F(%) was measured on approximately 20 g of pellets from the usable yield fraction, to which were added 40 g of 6-mm glass beads. After 30 min of blending in a 200-mL flask in a shaker–mixer (Turbula, GlenMills, Clifton, NJ) at 42 rpm, the mass retained on a 1400-μm sieve was weighed, and the friability F(%) was calculated according to the following equation:

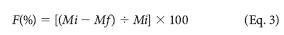
where Mi is the mass of granules before the test (i.e., 20 g) and Mf is the mass of granules retained by the sieve after the test. The test was performed in triplicate. The friability test showed the pellet surface's resistance to abrasion, which should be as high as possible to avoid abrasion during further processing.

The resistance to crushing R(N) was tested on 20 pellets of the usable yield fraction with a durometer (Computest, Kraemer Elektronik, Darmstadt, Germany). The diametral crushing force measured indicates the mechanical robustness of the pellets. It should be as high as possible to avoid pellet breakage during further processing.

Results and discussion

Design of experiments interpretation. The mathematical model generated for each response Y was a quadratic model with first-order interactions, designed according to the following equation:

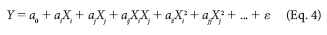
where X_i and X_j represent the levels of the factors; a₀ is the intercept representing the mean of the measured response data; a_i and a_j, a_ii and a_jj, and a_ij correspond to the coefficients of first-order terms, the coefficients of second-order quadratic terms, and the coefficient of second-order interaction terms, respectively. The coefficient corresponding to a factor or interaction shows its importance to the studied response. The symbol e represents pure error.

To simplify the design-of-experiments interpretation, the coefficients of second-order quadratic terms are not presented. Results are expressed according to the following equation:

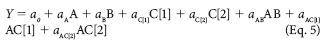
For the multilevel categoric factor C presenting three levels, the software calculated two coefficients, C(1) and C(2), corresponding to the difference between the high level and the average, and the difference between the low level and the average, respectively. The coefficient values were expressed in coded units to compare each one's relative effects to those of the others. Analysis of variance was performed to determine the significance of the model. A term that had a probability value lower than 0.05 was considered a significant effect. A probability value greater than 0.10 was regarded as insignificant. Table I summarizes the p values for the coefficients of factors for the different responses tested. Graphical analyses of responses were performed to determine the most important factors for a response by comparison with the other factors. Figure 4 shows the effects of factors and interactions on the responses. The red bars correspond to the factors that influenced the response. When an interaction between water quantity and extrusion system is underlined, the mean response value according to the extrusion system is shown according to three water-quantity values (i.e., minimum, medium, and maximum).

Table I: Significant effects for the main factors and interactions for various responses Yx.

Pellet characteristics. The pellet-morphology study showed great differences between the three extrusion systems. At the lowest water quantity (i.e., 22.5%), pellets created after radial extrusion presented a wide size distribution with many small particles, compared with pellets created after axial extrusion, which presented a majority of rods. Only dome extrusion provided pellets of the correct specifications. The pellets' morphology is linked to extrudate characteristics and the spheronization step. At low water quantities, radial extrudates were not densified enough and broke during the spheronization step, in contrast with axial extrudates, which were well densified but did not have enough plasticity to deform during the spheronization step. An increase in the water quantity improved the morphology of all pellets excepted those produced by the dome system.

The following equations obtained for pellet size, pellet dispersion, and usable yield, in which significant terms are shown in bold type, are represented in Figure 4:

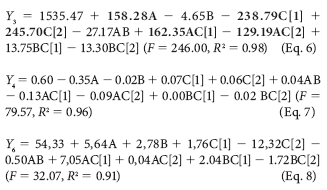
The design-of-experiments analysis showed that the three responses were significantly affected by water content and extrusion type. The usable yield fraction also was significantly affected by the extrusion speed. Pellet size and usable yield were more influenced by the extrusion system, whereas pellet dispersion was more influenced by the water quantity (see Figure 4). The pellet size was greatest in the axial system, moderate in the dome system, and smallest in the radial system. The dome system allowed the highest usable yield and the narrowest pellet dispersion, compared with the other systems. Increasing the water content increased the particle size and the usable yield, and decreased the pellet distribution. Other studies observed the favorable effect of water quantity on other extrusion systems, notably the pellet-size increase by agglomeration, the pellet-dispersion decrease, and the usable-yield increase (2, 5, 9, 24, 28, 29, 32). Moreover, extrusion speed increased the yield (14). These results can be explained by the previous morphological observations. At lower water quantities, the rods observed after axial extrusion did not pass through a 2000-μm sieve, and the fine pellets observed after radial extrusion passed through the 1400-μm sieve, which in both cases decreased the usable yield fraction. A significant interaction between the extrusion system and the water quantity showed that water quantity had less influence on pellet size in the axial system (but great influence in the radial system), and little influence on pellet distribution and usable yield in the dome system. Presenting a compromise between the three responses, the dome system offered the best characteristics in terms of pellet-size dispersion and usable yield fraction. At high water quantities, the three extrusion systems' results were more similar.

The following equation obtained for elongation is shown in Figure 4:

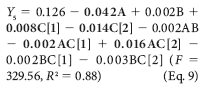
Pellet elongation was influenced significantly by water quantity and, to a lesser extent, the extrusion system. An increase in water quantity improved pellet morphology by decreasing elongation. In the same way, some studies showed that increased water quantity yielded better roundness (2, 9, 11). A significant interaction between the extrusion system and water quantity showed that dome extrusion gave the best characteristics in terms of the morphology of the granules at lower water quantities. Water quantity had less influence on pellet elongation for this system. For the axial system, the elongation (manifested by rods) decreased as the water quantity increased. For the radial system, the elongation at the smallest water quantity (manifested by nonspherical pellets) also decreased with an increase in water content. Finally, the extrusion dome produced the least elongation at the lowest water quantity and was not affected by an increase in the water quantity. At high water quantities, the three extrusion systems provided pellets with similar morphology.

Pellet yield fraction. The following equation obtained for friability is shown on bar graphs in Figure 4:

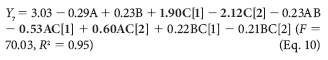
Pellet friability was influenced significantly by the extrusion system. The axial system produced the least fragile pellets, followed by the dome system. The radial extrusion produced friable pellets. The water quantity did not affect the friability significantly, but the authors observed an interaction between the extrusion system and water quantity. The increase in water quantity decreased the friability of pellets obtained after radial extrusion, did not influence the dome and axial systems.

Figure 4: The effects of factors and interactions on various responses (Y1–Y8).

The following equation obtained for diametral crushing force is represented on bar graphs in Figure 4:

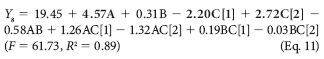
The pellets' diametral crushing force was influenced significantly by the water quantity and, to a lesser extent, the extrusion system. An increase in the water quantity improved pellets' resistance by increasing strong bonds, as other authors showed (24, 28). The axial extrusion system obtained the most resistant pellets, followed by the extrusion dome. The radial system yielded the least resistant pellets. These results can be explained by the differences in extrudate and pellet densification between the three extrusion systems. Combined with friability analysis, these results indicated that the axial system produced pellets with the best strength properties, followed by dome extrusion. The likely explanation is that extrudates and pellets obtained through axial extrusion are denser than those obtained with the other systems.

Figure 5: Response values according to extrusion method and water quantity for various responses (Y1–Y8).

Conclusion

The design of experiments showed significant differences between the three extruders on all the responses studied. Overall, the radial system produced the worst results for the drug product considered. Dome extrusion provided the best results in terms of productivity, pellet morphology, and pellet dispersion, and the axial system provided the best results in terms of pellets' mechanical properties. Pellet properties appeared to be linked to extrudate characteristics.

Water quantity had a favorable effect on all responses. An increase in water quantity improved the results obtained with the least efficient extrusion system and had a smaller influence on results obtained with the more efficient system. Water quantity had less influence on the dome and axial systems, which already yielded good results. A system that gives high-quality pellets over a wide range of watercontent values is less sensitive to water and more robust than one which produces high-quality pellets in a narrow range of water content. For the development of a formulation by an extrusion-spheronization process, a narrow water-level range could cause problems, especially for the scale-up phase of development. For this reason, the axial and dome systems are more appropriate than the radial system. The extrusion speed had no significant influence on pellet properties, but improved extrusion productivity significantly.

This study showed the particular advantages of dome extrusion in comparison with the two other systems. The results were nevertheless obtained for fixed spheronization conditions, which could be more appropriate for dome extrudates. It would be interesting to compare the three extrusion systems by testing various spheronization conditions. Moreover, results were observed for a highly soluble drug product at a fixed concentration in the formula. Testing different solubilities and concentrations of drug substances also could give complementary information about the efficiency of the three extrusion systems. The influence of formulation and spheronization conditions will be described in Part II of this article.

Amélie Désiré* is a doctoral student at École des Mines d'Albi-Carmaux and Centre de Recherche et de Développement Pierre Fabre, 3 ave. Hubert Curien, 31035 Toulouse Cedex 01, France tel. +33 0 5 34 50 62 79, fax +33 0 5 34 30 32 72, amelie.desire@pierre-fabre.comBruno Paillard is head of solid dosage forms, and Joël Bougaret is director of the Pharmaceutical Technology Department, both at Centre de Recherche et de Développement Pierre Fabre. Michel Baron is head of the Pharmaceutical Engineering Department at école des Mines d'Albi-Carmaux, and Guy Couarraze is head of the Pharmaceutical Physics Department at the Université Paris Sud.

*To whom all correspondence should be addressed.

Submitted: Aug. 4, 2010. Accepted: Sept. 30, 2010.

References

1. R. Gandhi, C.L. Kaul, and R. Panchagnula, Pharm. Sci. Technol. Today 2 (4), 160–170 (1999).

2. K. Umprayn, P. Chitropas, and S. Amarekajorn, Drug Dev. Ind. Pharm. 25 (1), 45–61(1999).

3. N.R. Trivedi et al., Critical Rev. Ther. Drug Carrier Syst. 24 (1), 1–40 (2007).

4. C. Vervaet, L. Baert, and J.P. Remon, Int. J. Pharm. 116 (2), 131–146 (1995).

5. J.J. Sousa et al., Int. J. Pharm. 232 (1–2), 91–106 (2002).

6. L. Baert et al., Int. J. Pharm. 86 (2–3), 187–192 (1992).

7. K.E. Fielden, J.M. Newton, and R.C. Rowe, Int. J. Pharm. 81 (2–3), 225–233 (1992).

8. J. M. Newton, S. R. Chapman, and R. C. Rowe, Int. J. Pharm. 120 (1), 101–109 (1995).

9. L. Baert et al., Int. J. Pharm. 99 (1), 7–12. (1993).

10. C. Schmidt and P. Kleinebudde, Eur. J. Pharm. Biopharm. 45 (2), 173–179 (1998).

11. E. Nürnberg and J. Wunderlich, Pharm. Technol. Eur. 11 (3), 30–34 (1999).

12. K. Thoma and I. Ziegler, Drug Dev. Ind. Pharm. 24 (5), 401–411 (1998).

13. K. Thoma, and I. Ziegler, Drug Dev. Ind. Pharm. 24 (5), 413–422 (1998).

14. E. Le Doeuff et al., 6th International Conference of Pharmaceutical Technology (Paris, 1992).

15. D. Sonaglio, B. Bataille, and M. Jacob, Pharm. Acta Helv. 72 (2), 69–74 (1997).

16. D. Sonaglio et al., Pharmazie 52 (2), 129–134 (1997).

17. D. Djuric et al., Eur. J. Pharm. Biopharm. 71 (1), 155–160 (2009).

18. A. Debunne, C. Vervaet, and J.P. Remon, Eur. J. Pharm. Biopharm. 54 (3), 343–348 (2002).

19. A. Dukić et al., Eur. J. Pharm. Biopharm. 66 (1), 83–94 (2007).

20. H. Kanbe et al., Int. J. Pharm. 337 (1–2), 56–62 (2007).

21. A. Dukić-Ott et al., Eur. J. Pharm. Biopharm. 70 (1), 302–312 (2008).

22. K.E. Fielden, J.M. Newton, and R.C. Rowe, Int. J. Pharm. 97 (1–3), 79–92 (1993).

23. E. Jerwanska et al., Int. J. Pharm. 121 (1), 65–71 (1995).

24. J.J. Sousa et al., Int. J. Pharm. 144 (2), 159–169 (1996).

25. L. Hellén et al., J. Pharm. Tech. Int. 4 (9), 50–60 (1992).

26. G.A. Hileman et al., Int. J. Pharm. 100 (1–3), 71–79 (1993).

27. C.C. Ku et al., Drug Dev. Ind. Pharm. 19 (13), 1505–1519 (1993).

28. B. Bataille, K. Ligarski, and M. Jacob, Pharm. Acta Helv. 65 (12), 334–337 (1990).

29. D. Sonaglio et al., Int. J. Pharm. 115 (1), 53–60 (1995).

30. K.A. Mehta et al., J. Control. Release 63 (1–2), 201–211 (2000).

31. S. Galland et al., Chem. Eng. Res. Des. 81 (9), 1237–1242 (2003).

32. S. Galland et al., Powder Technol. 157 (1–3), 156–162 (2005).

33. P. Kleinebudde and H. Lindner, Int. J. Pharm. 94 (1–3), 49–58 (1993).

34. D. Lutchman, C.M. Dangor, and D. Perumal, J. Microencapsul. 22 (6), 643–659 (2005).

35. C. Vervaet et al., Int. J. Pharm. 107 (1), 29–39 (1994).

36. C. Vervaet and J.P. Remon, Int. J. Pharm. 133 (1–2), 29–37 (1996).

37. R. Dietrich and R. Brausse, Pharm. Ind. 50 (10), 1179–1186 (1988).

38. A.M. Juppo et al., Eur. J. Pharm. Biopharm. 44 (2) 205–214 (1997).

39. P.J. Harrison, J.M. Newton, and R.C. Rowe, J. Pharm. Pharmacol. 37 (10), 686–691 (1985).

40. J.F. Pinto, G. Buckton, and J.M. Newton, Int. J. Pharm. 83 (1–3), 187–196 (1992).

41. L. Baert and G.R.B. Down, Int. J. Pharm. 107 (3), 219–222 (1994).

42. C. Lustig-Gustafsson et al., Eur. J. Pharm. Sci. 8 (2), 147–152 (1999).

43. G. Tomer, F.Podczeck, and J.M. Newton, Int. J. Pharm. 217 (1–2), 237–248 (2001).