Peer Reviewed: Numerical Simulation of Tablet Coating

Drum coaters are widely used in the pharmaceutical industry to produce film-coated tablets. The coating layer(s) around the tablet core can serve many purposes, such as tastemasking, coloring, control of the release of the API from the core of a tablet, application of an additional API, or protection of the tablet core from environmental influences. In this process, an atomizing nozzle above the tablets sprays the coating solution onto the tablets, which are mixed by the rotating drum. Heated air is forced through the system to enhance drying of the coating. In this way, each tablet receives a series of partial coatings (see Figure 1). A crucial issue is coating uniformity, which includes both inter-tablet uniformity (i.e., variation of coating mass from one tablet to the other) and intra-tablet uniformity (i.e., variation of the coating thickness and quality on the surface of a single tablet) (1–3). Inhomogeneity in coating thickness, for example, could lead to significant variations in API content or delivery rate. In many cases, a single tablet that fails testing can lead to rejection of the whole batch.

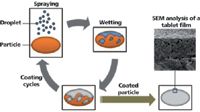
Figure 1: In the coating process, each tablet receives numerous partial coatings, which leads to a homogeneous coating layer. The scanning electron microscopy (SEM) picture shows a cross-section of the coating layer on the tablet core. (ALL FIGURES COURTESY OF THE AUTHORS)

Parameters that influence drum-coating performance can be divided into two groups. The first group includes parameters that can be adjusted by the operator (e.g., drum-rotation speed, fill level, spraying rate, or drying-air temperature). The second group are parameters that cannot be adjusted directly, but have a direct influence on the quality of the product (e.g., extension of the spray zone, mixing efficiency, air flow pattern, and quality of the coating spray) (4).

CHRIS ROGERS/GETTY IMAGES

Although drum coating is commonly used in the pharmaceutical industry, there have been relatively few scientific investigations reported in the literature. Moreover, process design has been based mostly on trial-and-error and operator experience. Recently, in addition to experimental work, advances in computational simulations have become an important tool for such investigations (5–8).

The major objective of this work is to show how a combination of different numerical techniques can help to provide a comprehensive, in-silico (i.e., via computer simulation) analysis of the whole tablet-coating process. The following aspects of the film-coating process aspects were identified (see Figure 2):

• Simulation of the tablet bed mixing using the discrete element method (DEM)

• Detailed numerical analysis of the interaction between spray droplets and the tablet surface by means of advanced multiphase computational fluid dynamics (CFD) methods

• Computational analysis of the coater internal flow in terms of drying air, spray, and wall effects using CFD.

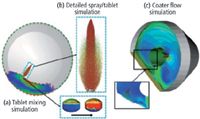
Figure 2: A schematic representation shows different process regimes of the coating process with an appropriate simulation approach used for each. For (a) and (c), colors denote velocity from slow (blue) to fast (red). In (b), the red color represents coating thickness; the tablet on the right in the lower box has a thicker coating.

The work aims to provide a deeper understanding of:

• The tablet-mixing process in an industrial pan coater, which allows optimization of the tablet residence time in the spray zone

• The local behavior of the spray droplets impacting on a single tablet, which leads to a wider understanding of film formation mechanisms

• The interaction between the spray and coater drying air, thus allowing a detailed analysis of the deposition efficiency of coating material on the tablet bed and insight into operative problems like overspray, filter plugging, or incomplete tablet-coat curing.

Computational simulation method

DEM simulations of non-spherical particles in different coater geometries were used to study the particle flow inside the coater and the residence time of the tablets in the spray zone (EDEM software v2.3, DEM Solutions). Material attributes of a sample placebo tablet were experimentally quantified as a control. DEM simulations consider the body forces and tangential contact forces on the tablets. Newton's equations are solved to calculate the velocity and rotational velocity of the tablet. Appropriate contact models, which are applied at the contact points of the tablets, account for tablet–tablet and tablet–wall collisions. A contact model based on the Hertz–Mindlin theory was used in this study.

Analyses of the local coating process and air- and spray-flow in the coating chamber were made using CFD multiphase simulations (FIRE v2009, AVL List). The software was used to study the interaction between the coating spray and the tablets, both locally (i.e., for a single tablet) and globally (i.e., by calculating the air flow in the whole coater). To this end, spray droplets were simulated with a discrete droplets method (DDM) Euler-Lagrange approach. Solvent evaporation is taken into account in order to estimate effects of drying-air flow. A two-dimensional model that incorporates sub-models for interfacial shear force, film evaporation, and heat transfer between the film, solid wall, and air describes the undried coating film.

DEM investigation of tablet movement

Figure 3 shows a DEM simulation for a continuous coater (Driaconti, DRIAM Anlagenbau). The tablets are colored according to their velocity, with the fastest near the top, at the upper part of the coater (red region). The spray nozzle is typically positioned in this region, because the tablets should move through the spray zone as fast as possible to eliminate over-wetting.

Figure 3: DEM simulations in a continuous-cycled coater (DriaConti, Driam). Tablet color indicates velocity from slow (blue) to fast (red).

Residence time of tablets in the spray zone is an important parameter. While residence time is difficult to determine from experiments, it is readily available from DEM simulation data. Figure 4 shows the fractional residence time (i.e., the residence time relative to the total time) for a simulation time of 60 s (9). Both tablet shape (quantified as length-to-height ratio) and fill level (ratio of tablet volume to total drum volume) influence the time that a single tablet spends exposed to the spray. To minimize coating variability or required coating process time, the fractional residence time should be as high as possible.

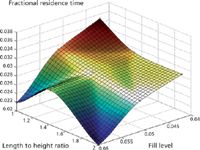
Figure 4: Average fractional residence time in the spray zone as a function of length-to-height ratio of tablet shape (round, bi-convex, and oval in increasing order) and coater fill levels.

In Figure 4, lower fill levels generally perform better than high levels, as is expected. The dependence of residence time on fill level, however, is not linear. Spherical and oval tablets show similar dependence on fill level. Bi-convex tablets, on the other hand, show a qualitatively different behavior; they are less affected by fill level and, therefore, outperform the other shapes at low fill levels.

Film formation

The Euler-Lagrange CFD simulations show how different physical parameters of the coating spray affect the coating process on a single tablet. The deposition behavior of the droplets on the tablet surface is analyzed in terms of film thickness and its homogeneity on the tablet. Simulations estimate the effects of tablet shape, droplet diameter, air temperature, spray rate of the coating, and water content of the coating solution on film formation. Simulation data allow analysis of important process characteristics such as behavior of the undried film on a tablet or the inter-tablet uniformity of the tablet film, measured as relative standard deviation (RSD), as a function of process parameters (see Figure 5). Small values of RSD translate to high uniformity. The surface plot in Figure 5 shows that lower atomizing pressure results in higher uniformity, but air temperature has little influence.

Figure 5: (a) In a schematic of the simulation approach (left), the colors denote water vapour content going from low (blue) to high (dark red). In the time (t) evolution of the film on tablets with different shapes (right), thickness is shown in shades of gray. (b) In a surface plot of intra-tablet coating uniformity for the bi-convex shape, smaller values mean better uniformity.

Spraying process in an industrial coater

CFD multiphase simulations were used to study the air and droplet flow inside a pan-coating device. This work simulated the effects of spray-gun position and injection angle on spray losses and coating efficiency.

Figure 6a shows the spray inside the coater after 0.5 s. Droplet color and size is proportional to the water content. The big droplets close to the spray nozzle had water content close to the initial spray-water fraction of 0.8. The small droplets at the right-hand side were already partially or completely dried. A fraction of the spray moved towards the tablet bed but was then dragged upwards, thus impacting the air inlet/outlet cylinder. The dry droplets followed the flow of drying air and left after a varying residence time. Figure 6b shows that the amount of spray loss varies for different spray nozzle positions. Positions 1 and 3 performed significantly better than the others, including the standard nozzle placement (B).

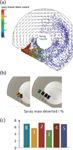
Figure 6: (a) Air flow and its effect on spray droplet movement. Flow direction is shown by gray arrows, the droplet diameter is drawn to scale, and the color of the droplets denotes the water content (i.e., blue droplets are already dried particles); (b) Effects of different spray gun positions and angles on the amount of spray loss (defined as the relative amount of mass that has deserted the system without contact to tablets).

Conclusion

Advanced numerical-simulation techniques can aid in the development and optimization of pharmaceutical tablet-coating processes. Investigating mixing performance and the resulting residence time distribution of different coating apparatus can be used to increase intra-tablet coating homogeneity. Numerical simulations of the interplay of drying air, spray, and tablet bed were used to examine both local and global coating performance. Computational results showed how the process parameters influence the film quality and coating uniformity. A usual rule-of-thumb is to place the nozzle at about one-third of the tablet bed, measured along the bed surface starting from the top. The study of different spray-nozzle setups showed that in terms of spray loss, following this rule indeed gave the best results. However, aiming the nozzle slightly away from the air inlet could reduce the amount of spray loss. Finally, inter-tablet coating variability was quantified by using the DEM method to determine the effects of tablet flow inside the coater in terms of residence time under the spray.

In addition to helping achieve a mechanistic understanding of the coating process, the computational data can be collected into in-silico design spaces, in which the correlation between critical process parameters and critical quality attributes provide an improved understanding of the design, optimization, and operation of industrial coating devices.

Gregor Toschkoff, Daniele Suzzi, PhD, and Siegfried Adam, PhD, are researchers at the Research Center for Pharmaceutical Engineering (RCPE) in Graz, Austria. Johannes Khinast*, PhD, is director of RCPE and head of the Institute for Process and Particle Engineering, Graz University of Technology, Inffeldgasse 21/a/II 8010 Graz, Austria, tel. +43 316 873 7978, khinast@tugraz.at.

*To whom all correspondence should be addressed.

Submitted: Apr. 15, 2011; Accepted: Jan. 16, 2012.

References

1. A. Kalbag and C. Wassgren, Chem. Eng. Sci. 64 (11), 2705–2717 (2009).

2. D. Suzzi, S. Radl, and J.G. Khinast, Chem. Eng. Sci. 65 (21), 5699–5715 (2010).

3. B. Freireich and C. Wassgren, Chem. Eng. Sci. 65 (3), 1117–1124 (2010).

4. S. Tobiska and P. Kleinebudde, Intl. J. Pharmaceutics 224 (1–2), 141–149 (2001).

5. L. Ho et al., J. Control. Rel., 119 (3), 253–261 (2007).

6. W.R. Ketterhagen, M.T. am Ende, and B.C. Hancock, J. Pharm. Sci. 98 (2), 442–470 (2009).

7. A. Alexander, T. Shinbrot, and F.J. Muzzio, Powd. Tech. 126 (2), 174–190 (2002).

8. G. Toschkoff et al., AIChE J. 58 (2), 399–411 (2012).

9. D. Suzzi et al., Chem. Eng. Sci. 69 (1), 107–121 (2012).