Process Stability, Performance, and Capability; What is the Difference?

Performance metrics for processes are an area of much regulatory interest currently. There isn’t always a readily available clear definition of what is needed, however, and guidance from regulators is not always consistent. This column goes back to the basics that were first set out by Shewhart (1) nearly 90 years ago and relates them to some modern process performance and capability indices.

Definitions are important in providing a consistent nomenclature, and the global International Organization for Standardization (ISO) standard 3534-2:2006 (2) will be used.

Process variation

All process measurement results are subject to variations that come from a variety of sources as was seen in the previous Statistical Solutions column (3). However, there are only two types as defined by Shewhart, namely common cause variation and special cause variation.

Common cause variation is the inherent noise in a process over time due to random effects and hence predictable within statistically derived limits.

By definition, a process that contains only common cause variation is said to be in statistical control.

Special cause variation occurs because of specific circumstances that are not always present manifesting themselves by, for example, a shift or drift in the process mean or excessive noise. If a process contains special cause variation, it is unstable from a statistical point of view, and the overall variation observed contains both common and special cause components. Control charts are designed to detect the presence of special causes of variation. The normal distribution is characterized by two parameters: a measure of location (the arithmetic mean or average) and a measure of dispersion (the standard deviation). An unstable process means that both of these parameters could be or are changing in an uncontrolled manner (Figure 1A) (4).

Figure 1: Process stability and capability (4). (Courtesy of the author)

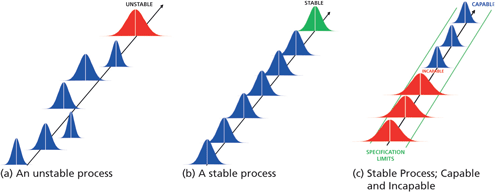
The task is to bring these two parameters into a state of statistical control. This would entail ensuring that the mean and the standard deviations were not varying significantly. This ideal situation is illustrated in Figure 1B. This process would then be said to be under statistical control (i.e., no special cause variation and stable common cause variation). In this state, the process is amenable to the tools of statistical process control (SPC). However, a stable process may not be statistically capable of meeting the specification limits. Figure 1C illustrates this, showing that the red process, albeit stable, is incapable. The desired state is to arrive at the blue capable state.

Capability is assessed using a family of quality metrics or indices called process performance and capability indices.

Quality metrics for process performance and capability

There are a variety of performance indices for processes in regular use. However, in this column, only four will be discussed, P_p, P_pk, C_p, and C_pk.

The definition and meaning of these four will be defined later. Of these four, only two have any practical relevance, P_pk, and C_pk. The other two are of theoretical interest as they do not occur in practice other than by chance. Process performance. P_p, a process performance index, relates to the output performance of a process, irrespective if it is in control or not, with the specification assuming that the long-term mean will be on the target for the product (an unbiased process).

The index is defined as a ratio of the difference between the upper and lower specification limits (called the specified tolerance in ISO) and the 99.73% probability of a value lying within ±3 standard deviations from the target (called the reference interval in ISO).

Hence, it can be said that this index would represent what the customer actually receives from the overall process (see Equation 1).

Equation 1.1

The overall standard deviation, S_t , is calculated from the usual formula for a sample standard deviation.

Equation 1.2

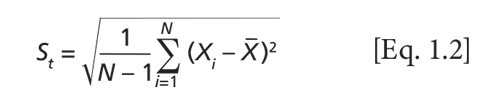
Where X is the mean of the N data points.

Values for P_p of 1.33 or more would indicate a highly capable process. A value of less than 1 would indicate an incapable process that would lead to out-of-specification (OOS) results.

As it is highly improbable that processes are unbiased, a practical process performance index would need to take this bias into account when assessing process performance. This is done by calculating the upper and lower process performance indices P_pkU and P_pkL using not the target but the actual observed mean to calculate them from:

Equation 1.3

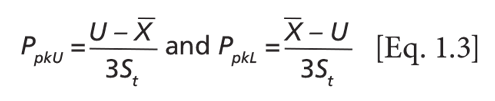
Hence the process performance index, Ppk is given by the smaller of the two values above.

Equation 1.4

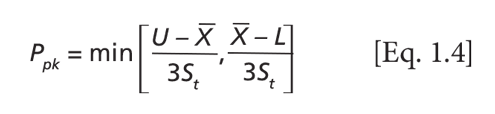
Process capability. Process capability refers to the performance of the process when it is operating under statistical control. Two capability indices are usually computed: C_p and C_pk in a similar way as was described with P_p and P_pk. However, C_p measures the potential capability in the process, if the process was centered, while C_pk measures the actual capability in a process, which is off-center or biased. If a process is centered, then C_p=C_pk.

Equation 1.5

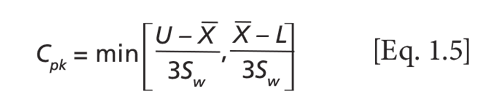
The critical thing to note is that while the formulae for P_pk and C_pk look very similar, the standard deviation used to calculate the reference interval for Cpk  is not S_t but S_w.

S_w is the within batch standard deviation (called the within sub-group standard deviation in ISO) not the overall process standard deviation. It is usually estimated from a Shewhart mean and range control chart using the formula:

Equation 1.6

Typical values for C_p  and C_pk are 0.5 to 1 for incapable processes, 1 to 2 for capable processes and >2 for highly capable processes.

A word of caution is necessary in interpreting C_pk values. C_pk analysis requires a normal underlying distribution and a demonstrated state of statistical process control. When reporting a C_pk value, a 95% or 99% confidence interval should always be reported because this takes into account the sample size used in the calculation (5,6).

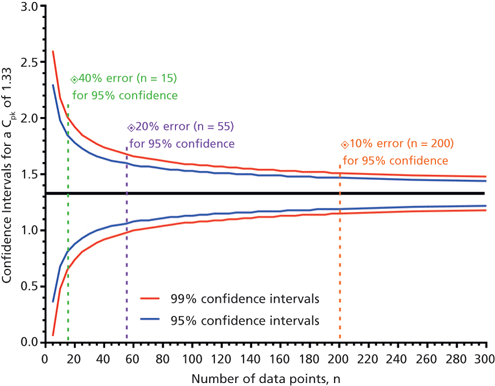
Sadly, this is usually missing. The confidence interval is extremely important because it is not always recognized that, for reasonably small confidence intervals around C_pk  values, the number of data points needs to be large. Figure 2 shows that to have a 95% confidence interval in C_pk  of 1.33 ±10% requires in excess of 200 data points. One commonly used approximation formula (5) for the confidence interval is:

Equation 1.7

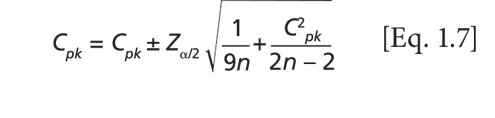
Hence the use of C_pk values for comparison of performance needs to be interpreted with great care when n is small.

Figure 2: Confidence intervals as a function of sample size for Cpk of 1.33. (Courtesy of the atuhor)

Conclusion

It has been shown how to differentiate between process performance and process capability. Equations, however, are not normally as clear as an example.

Figure 3 shows data from 157 batches of a product with a target of 7.0 and upper and lower specification limits ± 1.5. The data are nicely normally distributed as can be seen from the normal probability plot, but the long-term mean of 7.4 is biased high. However, the process capability C_pk is excellent at 1.31 and even with the bias would be unlikely to produce OOS results due to common cause variation (red curve).

Figure 3: Example of process performance and capability plots (Minitab 17). (Courtesy of the author)

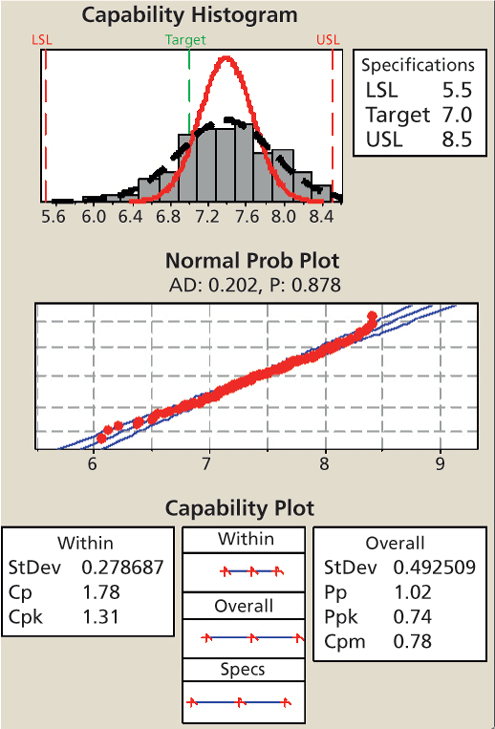
Unfortunately, the process suffers from considerable special cause variation, the dashed black curve, with P_pk  being an unacceptable 0.74 because the overall batch standard deviation (St ) is 0.49, whereas the within batch standard deviation (S_w) used to calculate C_pk is much smaller at 0.28. Note that if we could remove the mean bias, P_p would be a more acceptable 1.02. However, it would require a root cause investigation and process change(s) to remove some of the special cause variation(s) to approach a truly capable process.

References

1. W A Shewart, Economic Control of Quality of Manufactured Product (Van Nostrand, 1931).

2. ISO 3534-2:2006; Statistics-Vocabulary and symbols-Part 2: Applied statistics

3. C. Burgess, Pharmaceutical Technology 42 (5) 2018.

4. Redrawn & adapted from QMS – Process Validation Guidance GHTF/SG3/N99-10:2004 (Edition 2) Annex A, Statistical methods and tools for process validation, www.imdrf.org/documents/doc-ghtfsg3.asp.

5. ASTM E2281-15, Standard Practice for Process and Measurement Capability Indices.

6. S. Klotz and N.L. Johnson, Process Capability Indices (Chapman & Hall/CRC, 1993). 

Article Details

Pharmaceutical Technology
Vol. 42, No. 10
October 2018
Pages: 64-67

Citation

When referring to this article, please cite it as C. Burgess, "Process Stability, Performance, and Capability; What is the Difference?," Pharmaceutical Technology 42 (10) 2018.