News
Article
Pharmaceutical Technology
Establishing Blend Uniformity Acceptance Criteria for Oral Solid-Dosage Forms
Author(s):
This article introduces the concepts of pooled variance and the central limit theorem, which are intended for establishing acceptance criteria for blend uniformity data of granular powder blends when a significant degree of sampling bias is involved.
khemporn tongphay/shutterstock.com

With the current technologies for powder blend sampling, blend segregation during sampling (sampling bias) especially for granular blends will unavoidably occur, resulting in deviated (biased) blend assay results. This article introduces the concepts of pooled variance and the central limit theorem, which are intended for establishing acceptance criteria for blend uniformity data of granular powder blends when a significant degree of sampling bias is involved.
In 2013, FDA withdrew its draft guidance on blend uniformity (BU)-Guidance for Industry: Powder Blends and Finished Dosage Units--Stratified In-Process Dosage Unit Sampling and Assessment (1), in which Sections V and VII no longer represented the agency’s current thinking (2). Section V recommended taking at least three replicate samples from each of at least 10 locations within the blenders (tumbler mixers; or 20 locations in convective mixers). However, it only required that one sample from each location be tested to assess BU as part of first-stage testing, and the current FDA preference is to analyze all of the three replicates from each location (i.e., at least 30 blend samples at each of the three blending time points are tested) (2). It should be noted that the BU data for the three time points are intended to demonstrate the blending process robustness.
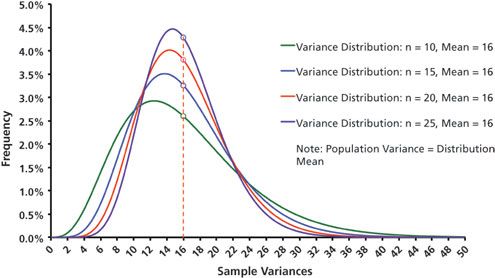
Figure 1: Sample variance distributions with population variance estimation.
As discussed in Bergum et al. (2), the use of nested sampling plans and testing of all replicate samples from each location allows the data to be subjected to variance component analysis in which the “between-location” and “within-location” variances are taken into account. The first type of variance is the variability across the sampling locations in a blender or during tablet compression and encapsulation processes, and the second is the variability between blend samples within a given sampling location.
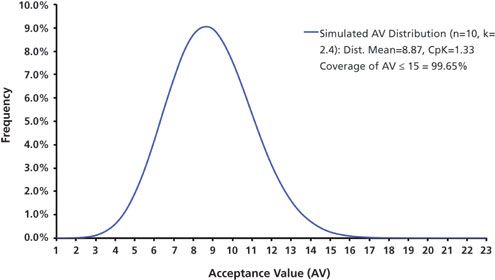
Figure 2: Simulated acceptance value (AV) distribution with coverage of AV ≤ 15 up to not less than (NLT) 99%.
High between-location variances will indicate non-uniformity within the blender (due to poor mixing) or reflect blend segregation during sampling (i.e., sampling bias) especially for granular blends. High within-location variances will indicate sampling bias (granular blends) or incomplete mixing during dosage forming steps such as tablet compression.
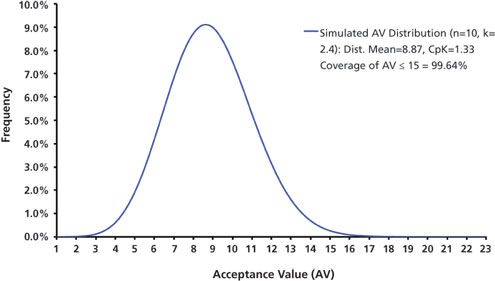
Figure 3: Simulated acceptance value (AV) distribution with coverage of AV ≤ 15 up to not less than (NLT) 99%.
With the current technologies for powder blend sampling, blend segregation during sampling (sampling bias) especially for granular blends will unavoidably occur, resulting in deviated (biased) blend assay results. In this case, it is no use evaluating the biased data using the variance component analysis approach.
This article introduces the concepts of pooled variance and the central limit theorem, which are intended for establishing acceptance criteria for BU data of granular powder blends when a significant degree of sampling bias is involved. FDA preference on testing all of the replicate blend samples (i.e., at least 30 blend samples) is still taken into account in this article.
Knowledge gained in the past
While taking blend samples by any sampling devices, the blend mass at the sampling location (i.e., in the blender) will be segregated due to the effect of the sampler movement. A blend is composed of the granules embedded with the active ingredient(s) and fine powders partly comprising lubricants and disintegrants; as such, the granular and fine powder portions are not always collected proportionally into the blend samples taken. Consequently, the overall blend uniformity in each of the blend samples may be diluted or concentrated, and therefore, be different from the true uniformity in the batch. Such a blend segregation caused by the sampling is referred to as sampling error or sampling bias resulting in biased mean and standard deviation values of the BU data. The magnitude of the bias may be extended such that non-normality of the BU data may occur.
CLICK TABLE TO ENLARGE Table I: Blend uniformity data of pre-mix and final blend samples (wet granulation in tablet product).
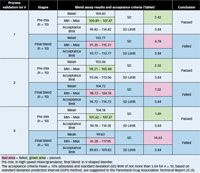
Actual case study data are illustrated in Table I where the pre-mix blend uniformity data are excellent due to the fact that the fine powder blends are marginally segregated while sampling. All the final blends (granular + fine powders) in the same process validation (PV) batches, however, will exhibit poor uniformity data due to the blend segregation as earlier discussed. However, in the tablet compression stage (of process validation batches), all the content uniformity and blend uniformity (i.e., weight-corrected) data are excellent. This observation implies that sampling at the final blend stage (granular) is biased.
CLICK TABLE TO ENLARGE Table II: Blend uniformity data of final blend samples (direct mixing in capsule product).

Another real case is that all fine-powdered materials (i.e., non-granular) directly blended for further capsule filling appear to have no sampling bias, as ilustrated in Table II. One of the lessons learned is that there is practically no sampling bias in fine powder blends, although a much higher degree of sampling bias exists in the granular blends. The acceptance criteria introduced in this article are intended for those granular blends where the blend sampling bias, especially at higher degree, is unavoidable.
The data of the two cases were evaluated because the compendial relative standard deviation (RSD) limit of not more than (NMT) 6% (n = 10) for content uniformity was official where the standard deviation prediction interval (SDPI) method was based on this particular RSD limit.
The pooled variance approach
Because variability of content uniformity (CU) data is influenced by a combination of those of BU and mass uniformity (MU) data, the approach to establishing the SD limit for BU data solely based on CU data variability such as the SDPI method is no more valid. In fact, variability of MU data should be taken into account. Such MU data would be available during the optimization phase in tablet compression or the encapsulation step. As an alternative, MU data may be obtainable upon using the placebo blends.
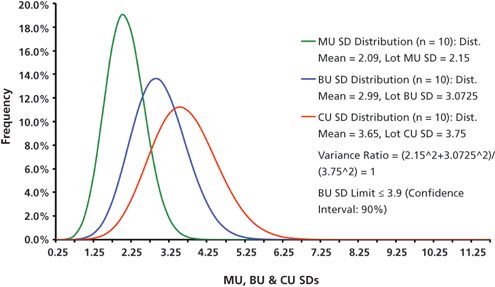
Figure 4: Simulated mass uniformity (MU), blend uniformity (BU), and content uniformity (CU) standard deviation (SD) distributions.
According to Sanford Bolton (4), the expression
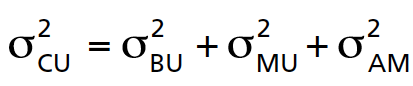
implies that CU variance is equal to the sum of BU, MU, and analytical method (AM) variances. Given that the AM variance is marginally low or usually determined as zero, Equation 1 is obtained:

where


Note that,
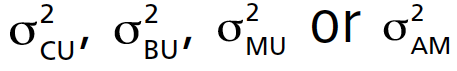
can be directly estimated by the average of multiple sample variance data (i.e., the pooled variance). Figure 1 illustrates and confirms that the means of sample variance distributions are equal to the population or lot variance.
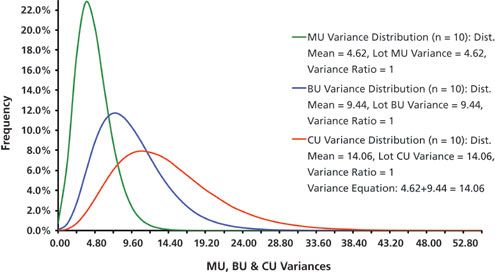
Figure 5: Simulated mass uniformity (MU), blend unifromity (BU), and content uniformity (CU) variance distributions.
When Equation 1 is rearranged, the expression is as follows (Equations 2 and 3):

In establishing the acceptance criteria limits for BU SD, it is necessary to pre-establish the lot CpK at 1.33 (i.e., lot SD = 3.75 [lot variance = 3.75^2 = 14.0625] using upper and lower specification limits [USL and LSL] of 115 and 85% label claim [LC], respectively) as the process baseline where it is found to provide the maximum acceptance value (AV) result marginally falling within the compendial limit of not more than 15. The simulated AV distributions demonstrating the justification of this particular baseline are illustrated in Figures 2 and 3 where the coverages for AV NMT 15 are at least 99%.
Figure 6: Simulated blend uniformity (BU), mass uniformity (MU), and content uniformity (CU) standard deviation (SD) distributions.
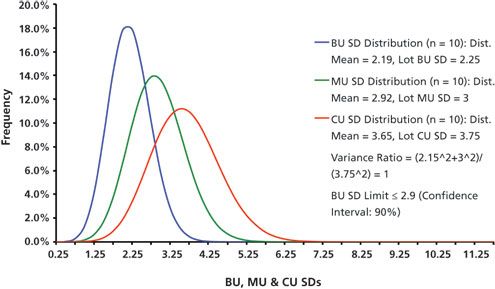
Two scenarios are introduced and discussed. Scenario 1 where lot MU SD < lot BU SD is illustrated in Figures 2, 4, and 5. Scenario 2 where lot MU SD > lot BU SD is illustrated in Figures 3, 6, and 7. From Figure 4, if lot MU SD is 2.15 and lot CU SD is fixed at 3.75 (from Equation 3 above), then lot BU SD is 3.0725 (calculated using Equation 3). The upper bound (UB) for BU SD (90% confidence interval) in tumbler mixers (n = 10) is 3.9 (in Excel, =3.0725*(CHIINV(0.1,10-1)/(10-1))^0.5). In convective mixers (n = 20), and the UB for BU SD is 3.7 using the same calculation method. So the BU SD limits will be 3.9 and 3.7 for n = 10 and 20, respectively. Now it can be seen that the BU SD limits are subject to variability of MU data. One can see that Equation 1 is proven by Figures 4-7 (i.e., where the lot CpK values for CU data are 1.33).
Figure 7: Simulated blend uniformity (BU), mass uniformity (MU), and content uniformity (CU) variance distributions.
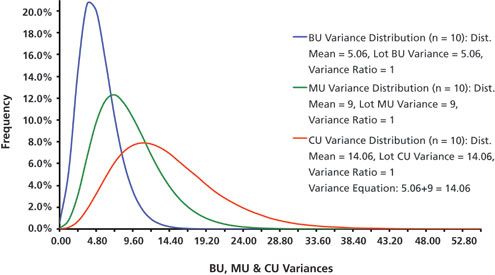
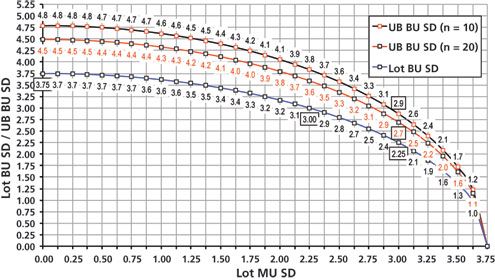
In Figure 6, the SD limits are calculated to be 2.9 (n = 10) and 2.7 (n = 20) which are also illustrated in Figure 8. Figures 5 and 7 illustrate the relationship between the sample variance distributions for MU, BU, and CU data. Figure 8 provides the relationship between the three SD data as a quick reference for BU SD determination.
Note that those BU SD limits are based on the assumption that the degree of blend uniformity in blending and compression or encapsulation steps remains practically the same (i.e., having no significant blend segregation).
Granular blend uniformity acceptance criteria
The central limit theorem in statistics states that the averages of non-normal data will normally distribute. By this rule, if the non-normal BU data fail to pass the acceptance criteria limits, the average data of at least two replicate samples at the same sampling locations should readily meet the limits.
Figure 8: Relationship between lot mass uniformity (MU) standard deviation (SD) vs. lot blend uniformity (BU) SD and upper bound for BU SD.
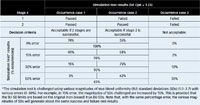
Based on the FDA recommended blend sampling plan, three replicate blend samples from each of not less than 10 locations are taken. The agency prefers that all the samples be tested and evaluated rather than one sample from each location as in the past. Based on the simulation test results (see Table III), the two-stage acceptance criteria, on which the decision criteria summarized also in Table III, are introduced as follows.
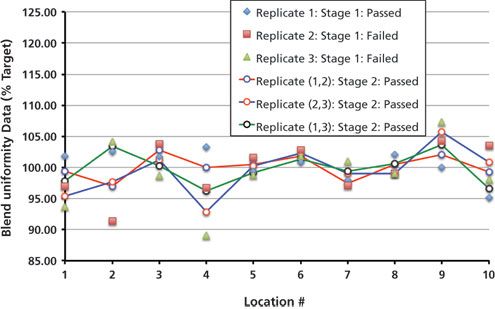
Figure 9: Plotted blend uniformity data (three replicate granular blend samples).
Acceptance criteria stage 1: For each of replicates 1, 2, and 3, blend data is evaluated.
- Mean ± 10% (absolute)
- SD ≤ P where SD is standard deviation for each replicate data and P is the calculated SD.
Acceptance criteria stage 2: For each of average data sets*, the average data of the blend samples at the same locations are evaluated (* i.e., three sets from replicates 1 and 2, 2 and 3, and 1 and 3).
- Grand average ± 10% (absolute), i.e., grand average for each average data set.
- SDaverage ≤ P where SDaverage is standard deviation for each average data set and P is the calculated SDaverage.
CLICK TABLE TO ENLARGE Table III: Decision criteria on meeting granular blend uniformity acceptance criteria.
A good practice in granular blend sampling should be taken into consideration so that the sampling is not too biased (i.e., the true blend data is not rejected by mistake). A scenario of granular blends is presented in Table IV and plotted in Figure 9 where locations 2 and 4, having wide spreads of BU data (12.76 and 14.23 ranges, respectively), are likely to create the non-normality that causes the failure in stage 1. In the scenario, the overall requirement (although failed in stage 1) is met and, finally, it passes the acceptance criteria.
Discussion
CLICK TABLE TO ENLARGE Table IV: Blend uniformity data (three replicate granular blend samples).

Based on the simulation test results in Table III, the various magnitudes of errors represent the various degrees of blend sampling errors. The tabulated test results (% occurrence) demonstrate justification of the decision criteria to accept the BU data and also confirm validity of the established acceptance criteria. From these particular test results, having the two-stage acceptance criteria seems to be discriminative enough to accept only the conforming BU data, leading to successful CU data (i.e., the non-conforming data are rejected). The average data for three replicates, if established as stage 3, would be too normalized, and the poor and non-normal BU data may be accepted.
To demonstrate the mixing robustness, blend sampling was performed on three different blending time points (i.e., a large number of up to at least 90 blend samples are taken and tested). The introduced acceptance criteria were also intended to handle a great number of data so that the failure result will occur only when the granular blending process is truly poor.
In case of fine powder blends, such as pre-mixes prior to wet granulation or direct blends prior to direct compression, there are also three replicate blend samples where each of the replicate data is required to meet the acceptance criteria in stage 1 (i.e., stage 2 is not necessarily applied to the fine powder blend data). It may be also required that the three replicate data (fine powder) are subjected to variance component analysis (5). The detail on this statistical tool is out of the scope of this article.
BU during tablet compression or encapsulation stage (i.e., available as weight-corrected data in process validation batches) may not totally reflect the true uniformity in the final blend stage because of blend segregation during the dosage forming steps. The data, however, is still useful to support that BU during the final blending step is at least not poor.
Essential elements for the acceptance criteria establishment include:
- FDA’s current thinking is taken into account (i.e., 30 BU data for 3 replicate blend sample data from at least 10 locations are evaluated).
- Experience and lessons learned show that granular blend data are readily non-normal because of a significant degree of sampling bias.
- For granular blends, the non-normal data needs to be handled and accepted by normalizing the data using central limit theorem method.
- The concept of pooled variances is introduced using the expression
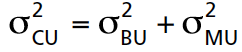
- as the science-based approach to acceptance criteria establishment by demonstrating the inter-relationship between
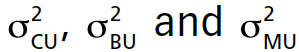
- to confirm that sample variance distribution means (for any sample sizes) are equal to the corresponding lot variance and also confirm that

- Process baseline is pre-established by using processes with lot CpK for CU data pre-set at 1.33 where the coverage for AV results NMT 15 is up to at least 99%.
- Simulation test challenged with sampling errors is performed to demonstrate and confirm that the acceptance criteria are justified and robust:
- Robustness of the acceptance criteria is demonstrated through challenging with different magnitudes of lot BU SDs with varied values of errors.
- Discrimination is built into the acceptance criteria using the two-stage acceptance criteria rather than the three-stage; and poor data will be rejected.
Conclusion
To meet the FDA’s current thinking, there may be several proposed options for BU acceptance criteria. The proposal in this article is scientifically based on process benchmarking (lot CpK at 1.33), central limit theorem, and pooled variance, which are supported by the simulation test results illustrated in Figures 2 to 7 and Table III. Such illustrations will demonstrate that meeting the established BU acceptance limits, especially for granular blends for oral solid-dosage forms, will guarantee with a high assurance (i.e., 90% confidence interval) that the CU data will pass the test at a high probability (i.e., at least 99% coverage for n = 10).
References
1. Center for Drug Evaluation and Research (CDER), Food and Drug Administration, Draft Guidance for Industry: Powder Blends and Finished Dosage Units - Stratified In-Process Dosage Unit Sampling and Assessment, October 2003 (withdrawn on August 7, 2013).
2. J Bergum et al., ISPE Pharmaceutical Engineering 34 (2) (March/April 2014).
3. PDA, Technical Report No. 25, Blend Uniformity Analysis: Validation and In-4. Process Testing, 1997, Volume 51, No. S3 (Supplement).
4. S. Bolton and C. Bon, Pharmaceutical Statistics: Practical and Clinical Applications, 4th Edition, (Marcel Dekker, NY, 2004).
5. D.C. Montgomery, Introduction to Statistical Quality Control, 6th ed.; (John Wiley and Sons, Hoboken, NJ, 2009).
Article Details
Pharmaceutical Technology
Vol. 41, No. 2
February 2017
Pages: 42–52
Citation
When referring to this article, please cite it as P. Cholayudth, “Establishing Blend Uniformity Acceptance Criteria for Oral Solid-Dosage Forms,” Pharmaceutical Technology 41 (2) 2017.
Newsletter
Get the essential updates shaping the future of pharma manufacturing and compliance—subscribe today to Pharmaceutical Technology and never miss a breakthrough.