News
Article
Pharmaceutical Technology
Calibration of instruments: Is Your UV Spectrometer Accurate Enough
Author(s):
This article takes a statistical look at the calibration requirements for a UV spectrometer.
All calibrations are subject to error. It is essential to ensure that qualified instruments are employed in the regulated environment. GMPs both in the United States and Europe require demonstrable control of instruments and systems. In the US, for example, under the cGMP regulations (1):
“Laboratory controls shall include: (b) (4) The calibration of instruments, apparatus, gauges, and recording devices at suitable intervals in accordance with an established written program containing specific directions, schedules, limits for accuracy and precision, and provisions for remedial action in the event accuracy and/or precision limits are not met. Instruments, apparatus, gauges, and recording devices not meeting established specifications shall not be used.”
The United States Pharmacopeia (USP) General Chapter <1058> on Analytical Instrument Qualification became effective in August 2008 (2). Analytical instrument qualification (AIQ) describes the framework and general activities necessary to ensure the suitability of an analytical instrument for its intended use.
Calibration has been defined as “the set of operations which establish, under specified conditions, the relationship between values indicated by a measuring instrument or measuring system, or values represented by a material measure, and the corresponding known values of a reference standard” (3). The question, therefore, is how good an agreement does there have to be between the measurement on a spectrometer with that certified on the reference standard certificate. This, of course, might be a simple statement of either an absolute difference or a percentage difference. This article looks at the issue from a statistical perspective.
Calibration of the absorbance scale of a UV spectrometer
A Certified Reference Material (CRM) for absorbance has been purchased, which has traceability to a National Measurement Laboratory (e.g., National Institute for Science and Technology [NIST] in the US). The spectrometer’s wavelength scale error is small enough not to cause any significant absorbance error at the wavelength of interest. Using the recommended operational procedures, six independent measurements of the CRM are performed at the wavelength of interest and the following absorbance data are obtained: 0.464, 0.468, 0.463, 0.465, 0.467, and 0.469.
From these six data measurements, a mean value of 0.4660 is derived. From the certificate of the CRM, the certified value is given as 0.4702. There is, therefore, a difference between the measured mean value and the certified value of 0.0042 absorbance units as illustrated in Figure 1.
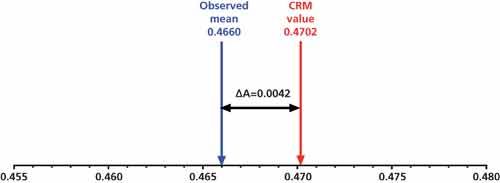
Is this difference acceptable? Is the difference statistically or analytically significantly different? Looking at the difference from a statistical perspective, noting that the 95% confidence interval,
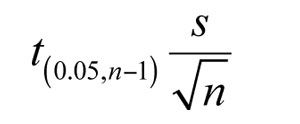
called the expanded uncertainty in ISO, the upper 95% confidence limit of the mean can be calculated,
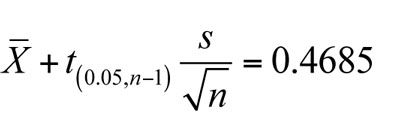
from the estimated standard deviation, s, which is 0.0024. The result is shown graphically in Figure 2.
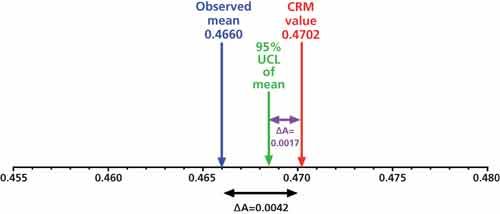
We can now see that at 95% confidence there is a difference of only 0.0017 absorbance units between the UCL and the certified value. Is this difference small enough that one can consider the absorbance scale sufficiently accurate?
The CRM has been certified to ISO standards (4) and, therefore, provides an expanded uncertainty for the certified value. In this example, the expanded uncertainty, U, is 0.0034, which corresponds to 95.45% confidence as the coverage factor, k, is 2 (5). The standard deviation of the CRM, therefore, is half this value and is 0.0017. Figure 1 can now be modified to show the calculated distributions for both the measurements and the CRM in Figure 3.
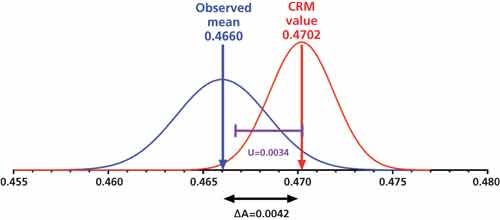
In Figure 3, it can be seen that the observed mean does not lie within the expanded uncertainty of the CRM. The cumulative probability of values can be calculated up to and including the mean value in the CRM distribution using, for example, MS Excel, with Equation 1:
Probability =NORM.DIST(0.4660,0.4702,0.0017,TRUE)*100 = 0.67 [Eq. 1]
(Note: This equation is from Excel in Office 2010 & 2013 where the main statistical functions have been updated. NORMDIST in previous versions will work also.)
It can be seen that there is only a less than 1% chance that the values up to the observed mean lie within the distribution of the certified value and also the mean lies outside the expanded uncertainty of the CRM. Should it be concluded that our spectrometer is inaccurate?
The answer is no because the CRM was measured on the same spectrometer and, therefore, the combined uncertainty needs to be calculated taking into account both error sources, the spectrometer’s and the CRM, in the overall uncertainty which is calculated from Equation 2:
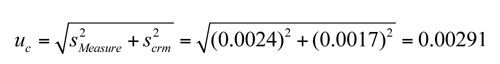
[Eq. 2]
For a coverage factor of k=2, the overall expanded uncertainty of our measured CRM is 0.0058.
Figure 3 can now be revised to show the effect of the combined uncertainty calculation in Figure 4. Note that the combined uncertainty distribution is broader than either the measured distribution or that of the CRM.
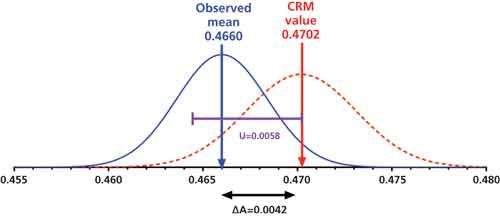
It is apparent now from Figure 4 that the observed mean lies not only within the expanded uncertainty of the measured CRM but also, if the earlier Excel probability calculation is repeated using the revised value for the standard deviation, uc
Probability =NORM.DIST(0.4660,0.4702,0.00291,TRUE)*100 =7.45% [Eq. 3]
There is now approximately a 7.5% chance that the cumulative probability of values up to and including the observed mean value lie within the modified distribution of the certified value of the CRM. It can, therefore, be concluded that the spectrometer is sufficiently accurate.
References
1. CFR 21 Part 211.160, General requirements
2. USP, USP 36-NF 31, General Chapter <1058> Analytical Instrument Qualification (United States Pharmacopeial Convention, Rockville, Maryland, 2012).
3. Glossary to European Union GMPs; accessed Dec. 6, 2013.
4. C. Burgess, Pharm. Techn., 37 (9), 62-64, 73 (2013).
5. S.L.R Ellison and A. Williams (Eds). Eurachem/CITAC guide: Quantifying Uncertainty in Analytical Measurement, (3rd ed., 2012), accessed Dec. 6, 2013.

About the Author
Chris Burgess, PhD, is an analytical scientist at Burgess Analytical Consultancy Limited, ‘Rose Rae,’ The Lendings, Startforth, Barnard Castle, Co Durham, DL12 9AB, UK;
Tel: +44 1833 637 446; chris@burgessconsultancy.com; www.burgessconsultancy.com
Newsletter
Get the essential updates shaping the future of pharma manufacturing and compliance—subscribe today to Pharmaceutical Technology and never miss a breakthrough.






